Removable Discontinuity How To Find : How To Classify Discontinuities - And lim_(xrarra)g(x) = l = g(a) so g is.
Removable Discontinuity How To Find : How To Classify Discontinuities - And lim_(xrarra)g(x) = l = g(a) so g is.. A function is said to be discontinuous at a point. A hole in a graph. Formally, a removable discontinuity is one at which the limit of the function exists but does not equal the value of the function at that point; Removable discontinuity give an example of a function $f(x)$ that is continuous for all values of $x$ except $x=2,$ where it has a removable discontinuity. I'd like to train my eye to better classify discontinuities, and i was hoping someone could offer a list of scenarios or perhaps a similarly you might find it fun to explore the asymptotic behaviour of the following
This may be because the function does. Such a point is called a removable discontinuity. Function f has a removable discontinuity at x=a if lim_(xrarra)f(x) = l (for some real number l) but f(a) !=l we remove the discontinuity at a, by defining a new function as follows: You'll usually find removable discontinuities in rational functions, and the removable discontinuity can usually be identified by factoring the numerator and denominator of the function and canceling like factors. How did you find the bonus questions?
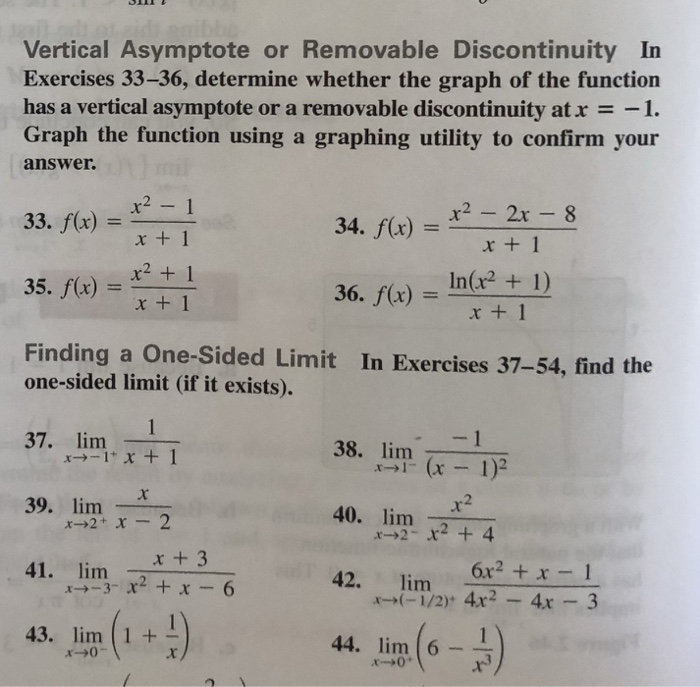
You'll usually find removable discontinuities in rational functions, and the removable discontinuity can usually be identified by factoring the numerator and denominator of the function and canceling like factors.
You'll usually find removable discontinuities in rational functions, and the removable discontinuity can usually be identified by factoring the numerator and denominator of the function and canceling like factors. The function is undefined at x = a. Mathematics · 9 years ago. For example, this function factors as shown The function f(x) is defined at all points of the real line except x = 0. This is the currently selected item. Explain how you know that $f$ is discontinuous at $x=2,$ and how you know the discontinuity is removable. Removable discontinuity give an example of a function $f(x)$ that is continuous for all values of $x$ except $x=2,$ where it has a removable discontinuity. G(x) = { (f(x),if,x != a),(l,if,x=a) :} for all x other than a, we see that g(x) = f(x). A function f(x) is said to have a removable discontinuity at x=a if: How do you solve a removable discontinuity? Surface area of a cylinder. Function f has a removable discontinuity at x=a if lim_(xrarra)f(x) = l (for some real number l) but f(a) !=l we remove the discontinuity at a, by defining a new function as follows:
The function f(x) is defined at all points of the real line except x = 0. In the graphs below, there is a hole in the function at $$x=a$$. Removable discontinuity a discontinuity is removable at a point x = a if the exists and this limit is finite. Points of discontinuity are also called removable discontinuities and include functions that are undefined and appear as a hole or break in the graph. And lim_(xrarra)g(x) = l = g(a) so g is.

At first i thought pole, order 2 but the discontinuity at $z=0$ turned out to be removable.
This may be because the function does. A hole in a graph. Please remove it if you find it inappropriate/wrong. A point of discontinuity is created when a function is presented as a fraction and an inputted variable creates a denominator equal to zero. They occur when factors can be algebraically canceled from rational functions. Notice that for both graphs, even though there are holes at $$x = a$$, the limit value at $$x how to use the pythagorean theorem. Which we call as, removable discontinuity. Discontinuities for which the limit of f(x) exists and is finite are called removable discontinuities for reasons explained below. F(a) could either be defined or redefined so that the new function is continuous at x=a. You'll usually find removable discontinuities in rational functions, and the removable discontinuity can usually be identified by factoring the numerator and denominator of the function and canceling like factors. Factor the numerator and the denominator. Explain how you know that $f$ is discontinuous at $x=2,$ and how you know the discontinuity is removable. A function is said to be discontinuous at a point.
You'll usually find removable discontinuities in rational functions, and the removable discontinuity can usually be identified by factoring the numerator and denominator of the function and canceling like factors. Drag toward the removable discontinuity to find the limit as you approach the hole. Points of discontinuity are also called removable discontinuities and include functions that are undefined and appear as a hole or break in the graph. G(x) = { (f(x),if,x != a),(l,if,x=a) :} for all x other than a, we see that g(x) = f(x). Learn how to find the holes, removable discontinuities, when graphing rational functions in this free math video tutorial by mario's.

So maybe instead removable_discontinuity=true a better name would be check_limit=true for a potential flag to add to solve().
A hole in a graph. Explain how you know that $f$ is discontinuous at $x=2,$ and how you know the discontinuity is removable. How do you solve a removable discontinuity? At first i thought pole, order 2 but the discontinuity at $z=0$ turned out to be removable. How did you find the bonus questions? How do you find removable discontinuities? Function f has a removable discontinuity at x=a if lim_(xrarra)f(x) = l (for some real number l) but f(a) !=l we remove the discontinuity at a, by defining a new function as follows: Notice that for both graphs, even though there are holes at $$x = a$$, the limit value at $$x how to use the pythagorean theorem. How do i find removable discontinuity of this function? Removable discontinuity a discontinuity is removable at a point x = a if the exists and this limit is finite. G(x) = { (f(x),if,x != a),(l,if,x=a) :} for all x other than a, we see that g(x) = f(x). Points of discontinuity are also called removable discontinuities and include functions that are undefined and appear as a hole or break in the graph. The value of the function at x = a does not match the…

Komentar
Posting Komentar